and the same, composited on a gray background:
(click for larger 4.7MB 1950x1635 .tiff image)
First of all, you need to look at Tamara Munzner's test images which are simple and clear. They're probably the best thing going.
And, here are newer versions of my hyperbolic/Euclidean/spherical images, along with small spheres occupying the same spaces -- one sphere at the center of each cell -- following suggestions from Tamara and Martin Bucher.
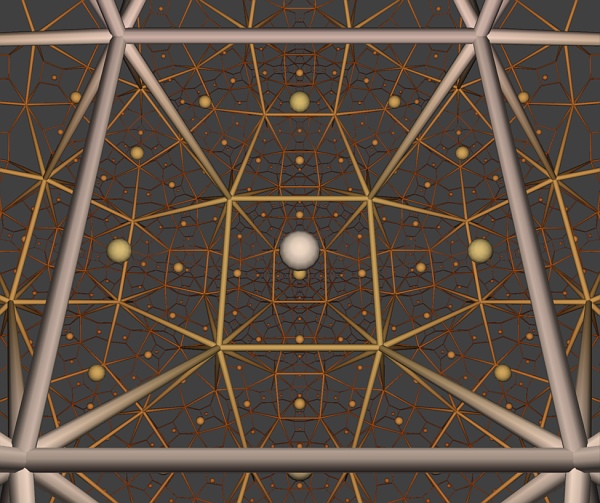
I'm including a gray-background version of the hyperbolic image on Tamara's advice, which does make the distant features stand out better. I personally prefer the black background, as it makes distant objects fade into obscurity -- which they really would do.
Note to Sci. Am. graphics people: the accompanying giant TIFF images have transparent backgrounds, so you can easily composite these images over any desired color.
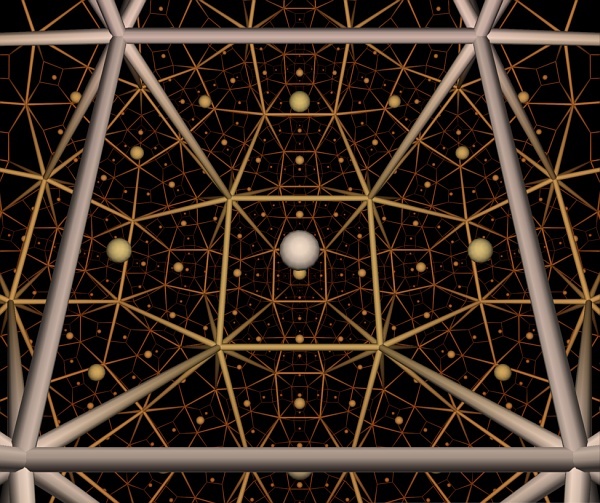
Here's a network of cube-like objects tiling hyperbolic space. Like ordinary Euclidean cubes, they have six identical faces meeting at 8 corners, with each face a regular 4-sided polygon. But their angles are more acute than Euclidean cubes could ever have: 5 of these shapes fit around each edge.
Notice how quickly the "pipes" at cube edges shink with increasing distance --
the most distant, deepest red features, at distance about 5 units, are almost
invisibly thin.

and the same, composited on a gray background:

(click for larger 4.7MB 1950x1635 .tiff image)
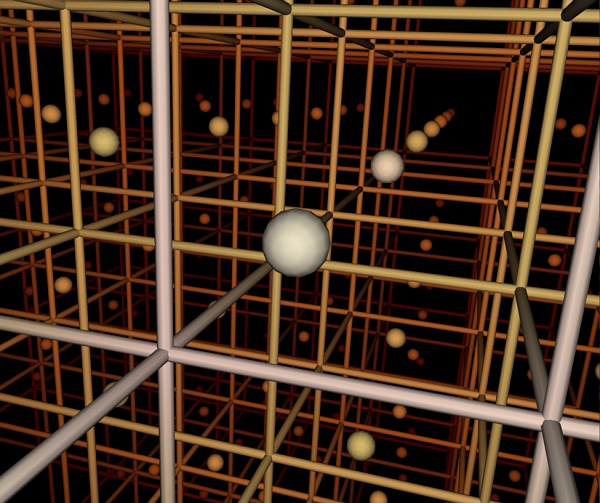
In contrast, here's a tiling of Euclidean space by cubes. They redden with distance in the same way as above, and the farthest of them are also around 5 units distant. But in Euclidean space, apparent size shrinks linearly with distance.
(click for larger 6.9MB 1950x1635 .tiff image)
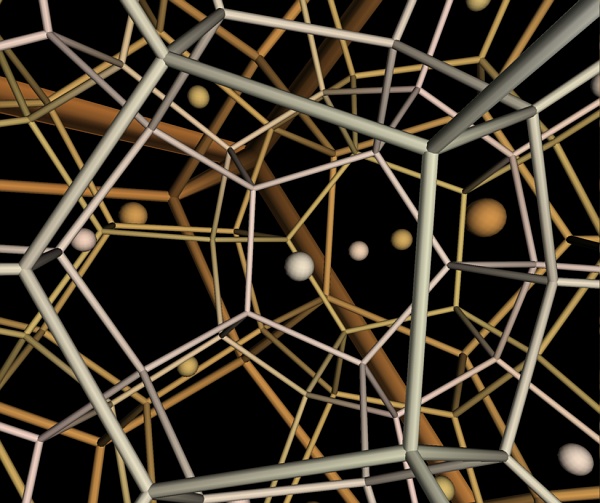
Here's a spherical space tiled by regular dodecahedra, three of which fit around each edge, with small spheres marking their centers. Apparent size in spherical space shrinks more and more slowly with distance, reaching a minimum at the yellow-white features lying 1/4 of the universe's circumference away. Between 1/4 and 1/2 the universe's circumference, apparent size increases, as shown by the chain of four spheres extending from center (nearest, gray) to center-right (farthest, brown). The deep reddish-brown dodecahedra appear largest in this picture, but they're also the most distant.
(click for larger 5.8MB 1950x1635 .tiff image)
Previous attempts live here.
Stuart Levy, slevy@ncsa.uiuc.edu.